Anomalous Interfacial Electron-Transfer Kinetics In Twisted Trilayer Graphene Caused By Layer-Specific Localization
- 10 Dec 2024
- Volume 27
- NANOscientific Magazine, FALL 2024
Kaidi Zhang, Yun Yu, Stephen Carr, Mohammad Babar, Ziyan Zhu, Bryan Junsuh Kim, Catherine Groschner, Nikta Khaloo, Takashi Taniguchi, Kenji Watanabe, Venkatasubramanian Viswanathan, and D. Kwabena Bediako*
This article has been condensed by NanoScientific from the original publication
in ACS Central Science, 2023, 9(6), 1119–1128, published on May 15, 2023, under
a Creative Commons Attribution 4.0 International License. This summary aims
to provide a shorter and more accessible version of the article. To read the full
original article, please visit https://doi.org/10.1021/acscentsci.3c00326.
ABSTRACT
Interfacial electron-transfer (ET) reactions are fundamental to converting electrical energy into chemical energy and vice versa. The electronic state of electrodes significantly affects ET rates due to variations in the electronic density of states (DOS) among metals, semimetals, and semiconductors. In this study, we demonstrate that by adjusting interlayer twists in well-defined trilayer graphene moirés, ET rates depend heavily on electronic localization within each atomic layer rather than the overall DOS. The high tunability of moiré electrodes results in local ET kinetics varying by up to three orders of magnitude across different three-layer configurations, surpassing rates observed in bulk metals. Our findings highlight that electronic localization, beyond ensemble DOS, is crucial for interfacial ET, offering insights into the high reactivity o¼en seen at electrode-electrolyte interface defects.
Introduction
Electron-transfer (ET) reactions at electrode-electrolyte interfaces are crucial for electrochemical energy conversion. The electronic structure of electrodes, described by theories such as the Marcus-Gerischer formalism and the Marcus-Hush-Christsey (MHC) model, influences ET rates. Recent studies show the importance of local density of states (DOS) in understanding interfacial ET kinetics, especially in semiconductor or semimetallic electrodes where atomic defects like vacancies and step edges enhance interfacial reactivity.
Azimuthal misalignment in atomically thin layers creates moiré superlattices, altering the electronic band structure depending on the interlayer twist angle. These structures, particularly at specific "magic" angles, form flat electronic bands with high DOS localized in real space. This has been observed in small-angle twisted bilayer graphene (TBG), where ET kinetics can be tuned by varying the twist angle (Ëm) between 0º to 2º.
The stacking order in multilayer graphene significantly affects its electronic properties. Bernal (ABA-stacked) trilayer graphene has dispersive bands, while rhombohedral (ABC) graphene has flat bands near the Fermi level, leading to correlated electron phenomena at low temperatures. Twisted trilayer graphene (TTG) structures, like monolayer-twist-bilayer (M-t-B) and A-t-A (alternating-twist-angle) heterostructures, exhibit extremely flat bands at magic angles around 1.5°.
These flattened bands create high DOS localized on AAB and AAA sites in M-t-B and A-t-A TTG, respectively, offering unique opportunities to study how electronic structure influences interfacial ET (Fig. 1). For example, larger DOS in A-t-A compared to M-t-B near their magic angles might correlate with higher ET rates based on the MHC model.
twisted trilayer graphene polytypes, with moiré wavelength ¹.
The blackparallelogram outlines the moiré unit cell in each case. (C, D) Computed local DOS (see Materials and Methods) for 1.2° M-t-B (C) and 1.5° A-t-A (D).
Results and Discussion
Sample Preparation and Characterization: Scanning electrochemical cell microscopy (SECCM) measurements were performed on both nontwisted (ABA, ABC) and twisted trilayer graphene samples, which were fabricated into devices (see Materials and Methods). Fig. 2A shows that naturally occurring ABA and ABC trilayers were mechanically exfoliated from bulk graphite and identified using optical microscopy and confocal Raman spectroscopy (see Materials and Methods and Supporting Information). The M-t-B and A-t-A TTG samples were prepared using the "cut-and-stack" method (see Materials and Methods), resulting in uniform twist angles (θm) of approximately 1.34° for the M-t-B device and 1.53° for the A-t-A device. The twist angle distribution and uniformity across the moiré samples were evaluated using piezoelectric force microscopy (PFM) (Fig. 2B) and scanning tunneling microscopy (STM) (Supp. Fig. 5, 10). The AFM images before and a¼er SECCM experiments show the trilayer graphene and hBN, with residues from the measurements clearly identified (Supp. Fig. 14).
Electrochemical Analysis: Cyclic voltammograms (CVs) measured with Ru(NH3)63+ showed that ABA domains exhibited sluggish electro-reduction rates, while ABC domains had more facile kinetics. Both TTG samples displayed highly reversible CVs with significantly higher rate constants compared to ABA and ABC graphene (Fig. 2C). Finite element simulations modeled the quantum capacitance (Cq) and double-layer potential (Vdl/Vapp) for various trilayer systems, revealing that flat electronic bands in TTG led to a more significant partitioning of the applied potential into Vdl near the charge neutrality potential (Fig. 3A, 3B). The θm dependence of the ET rate constant (k0) showed a strong, nonmonotonic variation with k0 ranging over two orders of magnitude (Fig. 3C).
Unexpected Trends and Structural Analysis: Despite higher DOS and Cq, A-t-A TTG exhibited lower k0 than M-t-B. B-t-M heterostructures also showed markedly lower k0 values than M-t-B, indicating that factors beyond ensemble DOS influence interfacial ET kinetics. STM analysis showed significant differences in the area distribution of stacking domains due to lattice relaxation, explaining the kinetic modulation observed at θm < 2° (Fig. 4G). Local DOS profiles showed that enhancements at AAB sites were localized on the top two layers of M-t-B, while DOS at AAA sites were localized on the middle layer of A-t-A. These differences in electronic localization correlated with the observed ET rate constants (Figs. 3C, 5A).
Implications and Future Work: Theoretical models based solely on θm-dependent DOS underestimated the experimental k0 values, suggesting the importance of interfacial electronic coupling, electric double-layer effects, and reorganization energy in ET kinetics. These findings highlight the significant role of electronic localization in interfacial ET and motivate further theoretical work to bridge the gap between theory and experiment, enhancing our understanding of ET processes in twisted trilayer graphene (Figs. 5C-E).
trilayer graphene. (A) Le: Optical micrograph of a device
fabricated from an exfoliated trilayer graphene flake
on hBN. Right: Confocal Raman spectra acquired in the
sites in A marked with red (ABC domain) and blue (ABA
domain) dots, along with the Raman map of the region
indicated with a yellow box in A. Scale bars: 10 μm. (B)
Le: Optical micrograph of an M-t-B device on hBN (Scale
bar: 10 μm). Right: A lateral PFM phase image over the
yellow boxed region in B reveals the moiré superlattice
pattern. Scale bar: 50 nm. (C) Representative steady-state
voltammograms of 2 mM Ru(NH3)63+ in 0.1 M KCl solution
obtained at ABA and ABC trilayer graphene, along with
1.3° M-t-B and 1.5° A-t-A, compared to that obtained at
an ~40-nm-thick platinum film. Scan rate, 100 mV s−1. The
inset illustrates the SECCM technique.
ET. (A) Calculated Cq as a function of the chemical potential
(Vq) for ABA, ABC, and TTG using the respective computed band
structures and DOS profiles (see Materials and Methods). (B)
Calculated fraction of applied potential on the double layer (Vdl/
Vapp) as a function of the applied potential (Vapp) for ABA, ABC, and
TTG. Vq and Vapp are relative to the charge neutrality potential.
Taken together, these data reveal that flat electronic bands
result in a more significant fraction of Vapp partitioning into Vdl
near the charge neutrality potential. (C) Dependence of the ET
rate constant, k0, on the trilayer graphene stacking type (ABA,
ABC) and θm for M-t-B TTG. Each marker denotes the mean of
measurements made on samples within a standard deviation
of the mean twist angle. The horizontal and vertical error bars
represent the standard deviations of θm and the standard error
of k0. The inset shows comparison of k0 values for M-t-B, B-t-M,
and A-t-A TTG at θm = 0.82 ± 0.05°.
(A, B, D, E) Constant-current STM images representative M-t-B (A,
B) and A-t-A (D, E) samples. Scale bars: 50 nm. (C, D) Qualitative
illustrations of different stacking domains in rigid and relaxed
M-t-B (C) and A-t-A (F) moiré unit cells. (G) Extracted area fraction
of different stacking domains in M-t-B TTG. The horizontal and
vertical error bars represent the standard deviations of θm and
the standard error of the area fraction, respectively.
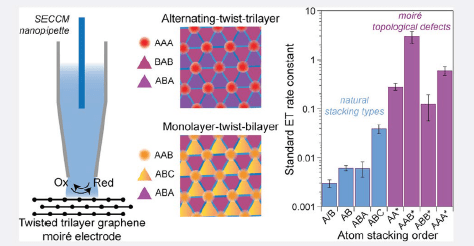
DOS localization. (A) Local standard Ru(NH3)63+/2+ ET rate
constants at few-layer grapheneindierentstackingconfigu
rations.“Artificial”moire-¾derivedstackingdomainsarelabeled
withanasterisk.Eachbaristhemeanlocalrate either measured
(for natural stacking) or calculated (for aritifical stacking)
for small twist angle samples. The error bars represent the
standard errors for the rates. (B) Schematic of M-t-B/B-t-M
graphene layers. (C) Layer-dependent DOS profile (see
Materials and Methods and Supporting Information Text) for
AAB stacking domains in M-t-B and B-t-M graphene at θm =
1.2°. Insets show real space DOS maps of each layer at ¿ = −3
meV. (D) Schematic of the A-t-A layers. (E) Layer-dependent
DOS profile for AAA stacking domains in A-t-A graphene at θm
= 1.2°. The insets show real space DOS maps of each layer at
¿ = −1 meV for θm = 1.2°.
used for area fraction analysis of different stacking domains. The blue
dash line was a line scan used for extracting AAB domain width. (B) Line
scan Z height over a horizontal distance. The purple line represents the
full-width-half-max cuto used for estimating the AAB radius.
Representative constant current STM images of various M-t-B in (A) - (E) and B-t-M in (F) of different twist angles.
dotted circles highlighted residues from the measurements. Both images were taken on a Park AFM NX10 system with non-contact mode with a set point of 10 nm. (C) Raman map overlayed with the traces of the trilayer graphene and hBN. The measurements on the black dotted circle
were used as rates on ABA graphene and those on the white circles were used as rates on ABC. Scale bar: 10 μm.
CONCLUSIONS
Controlling stacking geometries and twist angles in few layer graphene allows manipulation of standard ET rate constants over three orders of magnitude. Energetically unfavorable topological defects (AAA and AAB stacking domains), achievable only through the construction of a moiré superlattice, exhibit extraordinarily high standard rate constants due to moiré-derived flat bands localized in these defects. In addition to in-plane structural relaxation and electronic localization, the out-of-plane localization of the electron wave function on specific layers of twisted trilayer graphene results in measurable differences in ET rates at topological defects with different symmetries.
These findings demonstrate the sensitivity of interfacial ET kinetics to the three-dimensional localization of electronic states at electrochemical surfaces. They suggest that traditional measurements of ET rates at macroscopic electrodes might underestimate the true local rate constant, mediated by atomic defects that strongly localize electronic DOS at these interfaces. SECCM measurements prove to be powerful tools for probing layer-dependent electronic localization in atomic heterostructure electrodes.
Future experimental and theoretical work is needed to explore the microscopic origins of these electron-transfer modulations, considering reorganization energy, electronic coupling, and the electric double-layer structure. This work highlights the potential of moiré materials as a versatile and systematically tunable experimental platform for theoretical adaptations of the MHC framework, applied to interfaces with localized electronic states, representative of defective surfaces common in real electrochemical systems. In an applied context, twistronics is shown to be a powerful approach for engineering pristine 2D material surfaces to facilitate charge transfer processes with high kinetics, with implications for electrocatalysis and other energy conversion devices that could benefit from ultrathin, flexible, and/or transparent electrodes with high electron-transfer kinetics.
MATERIALS AND METHODS
Sample Fabrication:
Graphite and hBN were exfoliated using Scotch tape. Monolayer, bilayer, and trilayer graphene were identified using optical contrasts and confirmed by Raman spectroscopy. Trilayer graphene and TTG samples were fabricated using
the "cut and stack" dry transfer method on a temperature-controlled heating stage with an optical microscope and micromanipulator. Graphene flakes with both bilayer and monolayer parts were selected for monolayer twist bilayer or
bilayer twist monolayer samples. For a-twist-a samples, a large piece of graphene was cut into three pieces and transferred using poly(bisphenol A carbonate) film on PDMS.
Raman Mapping:
Confocal Raman spectra were recorded with a 532 nm laser at 3.2 mW, generating maps with a 2 μm step size. Spectra were fitted with single Lorentzian functions to differentiate ABA and ABC trilayers.
PFM Measurements:
PFM was performed on an AIST-NT OmegaScope with Ti/Ir-coated silicon probes. A 2 V AC bias at 820 kHz and a 25 nN force were used.
STM and AFM Measurements:
STM measurements were conducted using a Park NX10 STM module (Park Systems) at room temperature and atmospheric pressure. AFM measurements were taken by Park AFM NX10 (Park Systems) with non-contact mode with a set point of 10 nm. Pt−Ir tips were prepared by electrochemically etching 0.25 mm Pt−Ir wires (Nanosurf) in 1.5 M CaCl2 solutions. Scanned images were taken with a 0.2 V tip-sample bias and a 100 pA current set point. Twist angles of various samples were determined using Delaunay triangulation on the Gaussian centers.
Electron Microscopy Measurements:
TEM images of nanopipettes were obtained with a JEOL 1200EX microscope at 100 keV. Selected-area electron diffraction patterns were collected on an FEI Tecnai T20 S-TWIN microscope at 200 kV to resolve twist angles. Dark-field images were taken at the National Center for Electron Microscopy.
SECCM Measurements:
SECCM nanopipettes were made from single-channel quartz capillaries (0.7 mm inner, 1.0 mm outer diameter) using a laser nanopipette puller, producing 200 nm diameter pipettes confirmed by TEM. The surfaces were silanized, and filled with Ru(NH3)63+ or Co(phen)33+ solutions, and an Ag/AgCl wire was used as a quasi-counter reference electrode. Pipettes approached the target at 0.2 μm/s with a −0.5 V bias (0.5 V for Co(phen)33+), making contact at >2 pA or <-2 pA. After stabilizing for 30 s, cyclic voltammograms (CVs) were recorded by sweeping the potential at 100 mV/s for five cycles. For small twist samples (θ ≤ 0.15°) with moiré wavelengths >80 nm, only CVs from pipettes >200 nm were included. The pipette was retracted by 1 μm and moved horizontally for new measurements.
Chemicals, Finite Element Simulation and CV Fitting, Calculation of Band Structure and DOS:
Please refer to the original paper found at https://doi.org/10.1021/acscentsci.3c00326.
Supporting Information
The Supporting Information is available at https://pubs.acs.org/doi/10.1021/acscentsci.3c00326.
Category